Lesson 16- Earth Quakes, pH, and Decibel Scales

Earth Quakes - Richter Scale
4.0 » 5.0 = x10 times as much   
5.0 » 6.0 = x 10 times as much
4.0 » 6.0 = x 100 times as much

Rate= 10   P= 1


pH  scale:

              
R= 10  P= 1


Sounds Decibels:

 

40 dbs  > whisper      90 dbs > shout

           |  100000 times louder   |

R= 10      P= 10


Example 1:
How much more intense is an Earthquake of 6.8 to a 3.5?
F=IR ^ t/p                     M= magnitude   M=F       I= 1            t= change   
(delta)t= 6.8 - 3.5 = 3.3
M= 10^ 3.3/1
M= 1995.26

Example 2:
A chainsaw of 105dbs to a whisper of 40dbs
M= 10^ 65/10                      105-40= 65
M= 10^ 6.5
M= 3162277.66

Example 3:
Find the pH that is 80x more basic than water?
water= pH 7
M=R ^t/p
80= 10 ^t
log 80 = t log10                    *note log10 = 1
1.90= t
7+ 1.9= 8.9
t= 8.9
WATCH: when its basic you have to add the number to original equation, when it is acidic the number is the same

Lesson 15- Eponential Growth and Decay

In this lesson there are two different formulas:

The most common formula to use is:

F= IR^ t/p                    F: final amount       I: initial amount     R: rate of growth ( 5% = 0.05 +1 R>1)   growth decay ( 5%  R= 0.95 - goes down 5)     t: time       p: time for R to occur ( days, weeks, months,etc)  

* note: t and p must be in same units

The second formula is only used for continuous interest rates:

 P= Po e^ Kt          P: final amount       Po: initial amount      e: calculator function   K: growth/decay (no 1)  

t: time 

Example 1:

What will $3500 grow to, if invested at 6% interest for 10 years, compounded monthly?

F= IR ^ t/p               F=?     I= 3500   R= 1.005  t= 10    p=1/12   

(*note: if p is a fraction take the reciprocal and multiply it to the top number) 

F= 3500(1.005)^10/(1/12)

F= 3500(1.005)^120

F= $6367.88

Example 2:

What amount of money would grow to $ 4000 if invested at 91/4%, compounded annually for 4 years?

F= IR ^ t/p              F= 4000   I= ?   R= 1.0925   t= 4     p=1

4000   =     I  (1.0925) ^ 4/ 1

(1.0925)^4     (1.0925)^4

I= $2807.85

Example 3:

P= 100(0.87)^n represents the percent of caffeine in your body n hours after consumption. Write this equation as an exponential function with 1/2 as the base instead of 0.87

R= 0.87  (down) 13% per hr

0.5 = 0.87^ t                   

log0.5   =   t log0.87         1) log both sides

log0.87      log0.87

5  = t

Lesson 14- Logarithm Equations and Identities

Today's lesson had two methods for solving equations, log= # and log=log

Method 1: Log= #                                                                                                                                             

log2X = 3         1)  put into B^e = A form

2^3 = x

x= 8 

example2:

log3(x-4) = 2 + log3X

log3(x-4) = 2               1) put into B^e = A form

         x

3^2 = x-4              2) multiply both sides by x

            x

x(3^2) = (x-4/x)x       3) simplify

9x = x-4

8x= -4

x= -1/2  -- reject       

x= undefined 

*note: negative answers are tricky, they must become positive in original equation or are rejected.

       

Method 2: log = log                                                                                                                       

log5(x-3) + log5x = log5 10     1) create only one log

log5(x-3)x = log5 10            2) Drop logs

(x-3)x = 10

x^2 - 3x = 10

x^2 -3x -10 = 0

(x-5)(x+2)

x= 5, -2 -- reject ( negative does not work in eq'n.)

x=5

Identities:

Equation        vs.         Identity

2x+3 = 5                    x + x = 2x

x=1                            x = all real numbers

x= specific #                rejects allowed

Prove the identity and state the value(s) of x for which it is true:   ( make both sides equal)

  

logx + log(x +3) = log(x^2 +3x)

    LEFT SIDE           RIGHT SIDE

Left side:  logx(x+3)

               logx^2 + 3x = Right side

x must be great the 0     x>0

 

Lesson 13- Exponential Equations

In this lesson there are to different methods. Common base method, or the Log method

Method 1: Common Base

3^2x = 27^3x-1              1) Find Common Base
3^2x = (3^3)^ 3x-1
3^2x = 3^9x-3                2) Drop the Base
2x = 9x-3
3 = 7x                             3) Solve
3/7 = x

Check:  (put answer into original equation)
3^3/7 = 27^3(3/7) -1
2.55 = 2.55   
correct!!

Method 2: Logs

3^2x = 27^3x-1              1) log both sides
log3^2x = log27^3x-1
2xlog3 = (3x-1)log27            2) Factor out brackets
2xlog3= 3xlog27 - log27
2xlog3- 3xlog27= -log27        3) factor out x
x ( 2log3 - 3log27)  =      -log27                        4) Solve for x
    (2log3- 3log27)     (2log3- 3log27)
x= 0.42857
x= 3/7

*note: when using calculator, remember to use brackets or the answer may be wrong.

Lesson 12- Laws of Logarithms

Laws of logs:

loga(xy)= loga^x + loga^y
loga(x/y)= loga^x - loga^y
*note: when logs multiply they will add together, when logs divide they will subtract eachother

logaX^n= nlogaX
loga^n (square root) x = logax 1/n
logaB= logb/loga

Express 5 as a power of 2:


2^x= 5             
xlog2= log5           log both sides!
x (log2/log2)= (log5/log2)        isolate x (divide logs)
x= 2.32

Express as a single logarithm:

log8 - log5
= log 8/5          (subtraction=division)

logB + logD - 5logE - logA^2 + 1/2 logA
log= BxDxA1/2
         E^5xA^2
= log BD
      E^5 xA3/2

Write in terms of log x and log y:

log100x^2y
log100 + logx^2 + logy
2 + 2logx +logy

 

Lesson 11 - Exponentials + Logarithms

Exponentials vs. Logarithms:

Exponential is when you have an equation that looks like: 3^x
Logarithm is when you have an equation that looks like: log^x

The definition of a logarithm is with a exponent with a base of ten
logbA=E   or B^e =A they are the same but shown differently.
 b= base  a= answer e= exponent

Example:
loge 10  = 1
count the zeros to get the answer for any log with a base of 10

Exponential Form  vs Logarithm Form
3^4 = 81                     log381 = 4

the 3 is the base    4 is the the exponent
and 81 is the answer

Lesson 6 - Tools

The Absolute Value Tool:

How to change the graph of y=f(x) to y=| f(x) | -  For positive y- values, nothing changes on the graph. For negative y- values there will be a reflection over the x- axis.

example:

 1)  y= (x - 1)^2 - 2  - base graph                               All negative y-values become positive values

 2) y= | (x - 1)^2 - 2 | - reflection graph     

                                                          

 The Reciprocal Tool:

Steps:                                                                  

1. Base
2. Vertical Asymtotes (zeros)
3. Invariant points
4. Big points to small points
5. Small points to Big points

Lesson 5 - Combinations

Combination = Reflections, Shifts and Dilations
 Analyze:

If you have a dilation or reflection with a translation » Factor it!  
  example: y= square root of -2x   this equation would reflect over y-axis and a hor. comp by 1/2
 y= square root of 2x + 6 this equation would have to be factored out making it y= square root of 2(x + 3) with a hor. comp. by 1/2 and it would be shifted to the left by 3
  note: do stretches/ reflection first then do slides (translations)

List the transformation:

y= 1/2f(-3(x + 1)) -4    Vertical: comp by 1/2 and down 4   Horizontal: reflecting over the y-axis. comp by 1/3, left 1

Lesson 4 - Dilations

y = f(x) which goes to ky= f(x)

The graph will stretch or compress by 1/k
The graph compresses if the number is whole and stretches if it is a fraction.
This will make stretch/ compress vertically


y= f(x) which goes to y= f(kx)

The graph will horizontally stretch or compress by 1/k

examples:
y= f( 1/2x)   there will be a hor. stretch by 2
2y= | x |  divide by 2, giving you y= 1/2 | x | which means there will be a vertical comp. by 1/2

NOTE:  Horizontal compression or stretch deals only with x  and Vertical compression or stretch will deal only with y. Compression is a fraction and Stretch is a whole number.

Lesson 3 - Reflections

Rules for x and y axis:

 For y= f(x) to y= f(-x) , the graph of f(x) is reflected over the y-axis. Algebraically, x is replaced with - x.

 For y= f(x) to -y + f(x), the graph will reflect over the x-axis. Algebraically, y is replaced with -y.


 Relations between basic equations:

y= (-x)^2  is the same as y= x^2 but will reflect over the y-axis.
y= -(x^2) is the same as -y= x^2 and will reflect over the x-axis.
y= 1/ -x is the same as y= 1/x and reflects over the y-axis.
y= square root of 16 - x^2 which reflects over the x-axis.

Inverses:

An inverse is the reflection across the line y = x
Rules:
1) swap x to y
2) isolate y
3) rewrite f ^-1(x)

The notations:
y= f(x)  goes to y= f ^ -1 (x)

example: 
f(x)= -1/2x + 3
Find f^-1(x)
x= -1/2y +3
multiply everything by 2
2x= -y + 6
y= -2x + 6
f^-1 = -2x +6

Lesson 2 - Translations

Horizontal Translations (x) :

 y= f(x)   vs.  y= f(x -k)
For y= f(x) goes to y=f(x-k), the graph  of f(x) shifts to the right by k if k>0 and shifts to the left by k if k<0. How I remember this is if the equation is (x - k) than you shift the graph to the right because it becomes positive, and if the equation is (x + k ) than you shift the graph to the left because it becomes negative. With Horizontal Translations the graph will only move to the left or right.

example: y= | x |                                                    y= | x-3 |

  
                                       

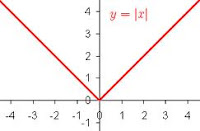
the first graph is the base and the second graph moved to the right 3.




Vertical Translations (y):

 y= f(x) vs. y- k = f(x)

 For y= f(x) goes to y - k=f(x), the graph of f(x) shifts up by k if k>0 and shifts down by k if k<0. How I remember this is if the equation is y + 3 = f(x) than you shift the graph down because it becomes negative, and if the equation is y - 4 = f(x) than you shift the graph up because it becomes positive. If the equation is y= f(x) +3 it will shift up by 3, and if the equation is y=f(x) -4 the graph will shift down by 4

example:   y = x^2 (solid line)   and  y = x^2 +1(dotted line)





     The solid line graph has stayed in its normal position but the dotted line has moved up 1 space.


Both Translations:
When you have both of the translations together you must do both translations.  I see it as if a number is grouped with the x like (x - 6) than I know you move the graph to the right by 6. If the equation is y -3 = (x - 6) than I know you have to move the graph up 3 and to the right 6.

Lesson 1 - Review

In lesson 1, we went over review from Math 11.


We went over functions you should know, here they are:

 LINEAR:
 y= mx + b    m being the slope       b being the y-int
ex: y= -1/2x + 2     -1/2 = m        +2 = b


QUADRATICS:
quadratics have 2 x's which makes a parabola shape, kinda like a  U, also will have only two x terms (zeros) on the graph.

as an example a quadratic formula would look like y= ( x + 2)^2 -1


CUBICS:

cubics  have three x's which make up a shape kind of like a N, and also means that the graph will only have 3 zero terms. This would make the graph "odd" meaning it will either start positive and end negative or the opposite.  ex: y= (x +2)(x-3)(x-4) or y= (x +1)^2(x-2)


QUARTICS:
 quartics have four x's, they are "even: meaning the graph will start at the top and end at the top, or start at the bottom end at the bottom, making either a M or W shape.
ex: y= (x+1)^2 (x-2)(x-3)

RECIPROCAL:
reciprocals have a vertical asymptote, which is where the graph will get closer and closer but never touch.
ex. y= 1/ (x-2)